Hi Steemians, today another story about two of my greatest loves cake and math!

Mister Cantor is the proud owner of the infinity patisserie which is located in the center of the city Mathematica. It is popular for its Koch flake cookies, Menger sponge cake and Feigen-baumkuchen. On Wednesdays, the patisserie chef Cantor produces a most delicious one meter long strawberry cake. From the top it looks something like this

Observe that Cantor placed small notes to indicate the edges of the cake. 0 is the left edge and 1 is the right edge. So a position on the cake, which is referred to as a cake-position, can take any value between 0 and 1. The cake is described by its edges. So we will denote the whole cake as an interval: [0,1]. The customer can decide how much of the cake he/she wants and Cantor will cut it accordingly.

The first customer

This Wednesday Cantor's first customer is a tall gentleman. "I would like to buy 0.8 meter of your strawberry cake. But the side of the cake is always a bit dry. Would it be possible to get a 0.8 meter middle part?"

"Yes, the customer is king after all!" Cantor replies. So Cantor cuts the cake, puts the cake in a nice box, which then goes into a nice big bag which results in one overjoyed customer. After the customer has left Cantor places two additional small notes to indicate the edges of the resulting two cakes:

A piece of cake is described by the location of its edges. So we denote a cake piece in interval notation. The cake intervals which are left are then described by [0,0.1] and [0.9,1]. So in total Cantor has sold 0.8m. In cake-position notation this corresponds to all the cake-positions for which the first decimal after the dot is a 2,3,4,5,6,7,8.

More enter

Shorly after, a married couple enters. "We would like to buy two pieces of 0.08 meter. But could we have the middle part?" the wife asks.

"Yes, of course!" Cantor replies. He cuts the four pieces, packages everything and two satisfied customer leave his patisserie. Cantor then places notes to indicate all the edges:

So the cake intervals which are left are [0,0.01], [0.09,0.1], [0.9,0.91] and [0.99,1]. In total Cantor has sold 0.8 + 2 x 0.08=0.96m. In cake-positions this corresponds to all cake-position for which the first or second decimal after the dot is a 2,3,4,5,6,7,8.

Now a family of four enters. "Could we get four pieces of 0.008 meter of the middle parts?"" the husband asks.

"Yes, no problem!" Cantor replies. He cuts four pieces, packages them and a happy family of four leaves his shop. Unfortunately, the pieces became too small to write clear notes. But the cake now looks like this:

So the eight pieces which are left are given by the intervals [0,0.001], [0.009,0.01], [0.09,0.091], [0.0999,0.1], [0.9, 0.901 ], [0.909 ,0.91], [0.99,0.991 ] and [0.999 , 1]. In total Cantor has sold 0.8 + 2 x 0.08+4 x 0.008=0.992m. In cake-positions this corresponds to all cake-position for which the first, second or third decimal after the dot is a 2,3,4,5,6,7,8.

Next 8 customers enter who each want a 0.0008 piece. And yes, they only want the middle parts. In total Cantor has sold 0.8 + 2 x 0.08+4 x 0.008+8 x 0.0008=0.9984m. In terms of cake-position he has sold the cake-positions for which the first, second, third or fourth decimal after the dot is a 2,3,4,5,6,7,8.

Next 16 customers enter who each want a 0.0008 piece. And again, only the middle parts of what is left. Then 32 come in who only want a 0.00008 piece of the left middle part pieces. This continues the whole day. In the city of Mathematica the day takes an infinitely long time. At the end of this day Cantor calculates how much cake he has sold:

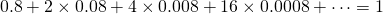
He concludes that he has sold the whole cake (see the technical appendix for the proof)! In terms of cake-positions he has sold the cake-positions for which any number after the dot is a 2,3,4,5,6,7,8.

Left-overs?

When Cantor looks over to the cake he can see that there is still some left. "Well I did never cut the cake's edges. So probably what is left are the cake edges." he mumbles to himself. Cantor realizes that all cake edges have the property that they can be written as a whole number times 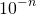for some whole number n. For example, 0.999 is a cake edge and it can be written as 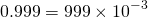. But this also means that the cake position 1/11=0.090909090... is not a cake edge since it cannot be written as a whole number times 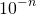. But Cantor has sold the cake-positions for which any number after the dot is a 2,3,4,5,6,7,8. The number 1/11=0.090909090... has no 2,3,4,5,6,7,8 appearing in it. Since it cannot be a cake edge it is still inside the cake! He exclaims:

" I am left with a paradoxical dessert!"

Photo by Laika AC

---

Conclusion/Reflection

Let us call the cake obtained after cutting away infinitely many pieces the Cantor cake. I derived the paradoxical property that altough the cake was originally 1 meter, when cutting away pieces with a total length of 1 meter to get the Cantor cake you end up with something that is more than just points.

What is the intuition behind this?

Before we can answer this you first need to realize that after having removed infinitely many pieces you do not end up with a collection of intervals. To see this observe that 0 must be an edge of the Cantor cake well what is the smallest edge greater than 0? You might say 0.0001 is a small edge point, but it is not the smallest since 0.00001 is also a edge point. Is this the smallest? No, since 0.000001 is smaller and again this is not the smallest. So you cannot think about the Cantor cake as something which is equal to a collection of intervals. Informally, you can describe it as follows

altough the Cantor cake is constructed by removing cake-intervals it is not equal to a collection of intervals. Thus, it transcends the natural intuition that we have for intervals.

When dealing with the Cantor cake you cannot rely on your intuition anymore since it is not in the realm of ordinary logic. And that is why you need the power of mathematics to lead you the way.

---

Further reading/sources

The mathematical object that this cake corresponds to is called the Cantor set. It has many more counter intuitive properties. Conventially, the Cantor set is explained in base three (counting when you have three fingers) but I did it in base ten since it does not require any knowledge about number bases. However, the theory for the base three Cantor set is identical to the base ten Cantor set since there is way to transform one into the other. If you want to find a more formal approach to Cantor sets you can have a look at the wiki-page over here. You can also checkout this book Understanding Analysis by Stephen Abbott (Springer, 2002).

The top photo is by Hedwig Storch

The figures where made using inkscape and all the equations were written using quicklatex. They are both free to use!

---

Technical appendix

The sum can be written as

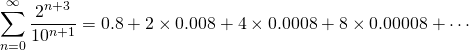
as is made clear by the right hand side. This is a geometric sequence which is equal to one. For the wiki click here

---

Thank you!

Thanks for being so kind to read my post. You are awesome! Please follow me if you enjoyed it. If you have any questions just post them below and I will answer them. Or if you might have a nice topic you want me to cover also let me know below. :o)

---

Owl tax

Source